All the fundamental interactions (electromagnetism, weak and strong nuclear forces, gravitation) are described by " gauge theories ", i.e. theories that are invariant to transformations depending on arbitrary functions of time, implying redundancy in their physical formulation. Only gauge-invariant quantities are observable.
This redundancy in the physical description leads to difficulties in the quantum formulation, where control of gauge invariance is more difficult to master.
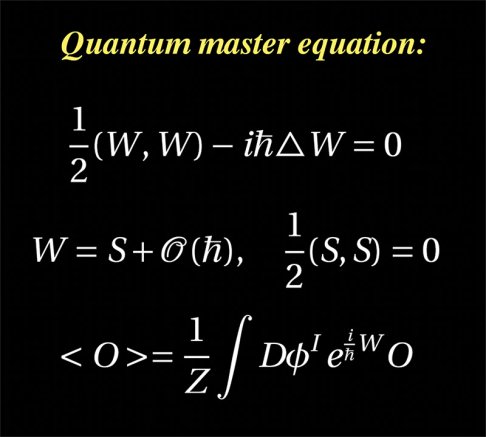
One approach that overcomes these difficulties is the anti-fields approach, developed by I. Batalin and G. Vilkovisky and for this reason also called the " BV approach".
This approach reformulates gauge invariance in algebraic terms by introducing new variables, the "ghost" fields (which appear only in intermediate states) and a zero-square differential operator, the " differential BRST " (Becchi-Rouet-Stora-Tyutin). Physical observables are given by the BRST differential cohomology classes in degree zero, with anomalies appearing in degree one.
The anti-field formalism not only provides a check on the gauge invariance of quantum theory, but has also proved to be very powerful, somewhat unexpectedly, already at the classical level (e.g., consistent classical deformations of gauge theories, connection with Gerstenhaber algebras, supervarieties etc.).
The lecture will be devoted to the systematic construction of the antifield formalism, general theorems on BRST cohomology and the calculation of this cohomology for Yang-Mills theories. The last lesson will be devoted to some modern developments.
The lecture will be followed by a colloquium :
" Topics in Quantum Gravity " on June 20 and 21 .
